|
REDOX POTENTIALS AND SIMPLE TEST TUBE REACTIONS This page explains how standard electrode potentials (redox potentials) relate to simple and familiar reactions that can be done in test tubes. | ||
|
Important: If you have come straight to this page via a search engine, you should be aware that this is just one page in a linked series of pages about redox potentials. You will find it much easier to understand if you start from the beginning. Links at the bottom of each page will bring you back here again. It is also important that you understand about redox reactions - particularly building ionic equations from electron-half-equations. Follow this link if you aren't confident about this, and use the BACK button on your browser to return to this page. | ||
|
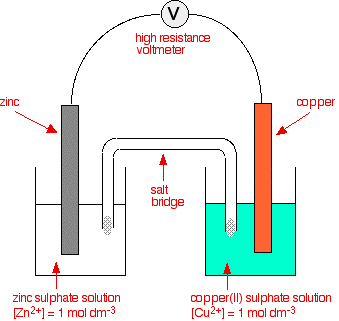
Combining a zinc with a copper half cell So far in this series of pages, we have looked at combinations of a hydrogen electrode with the half cell we have been interested in. However, there isn't any reason why you can't couple any two half cells together. This next bit looks at what happens if you combine a zinc half cell with a copper half cell. In the presence of a high resistance voltmeter
The two equilibria which are set up in the half cells are:
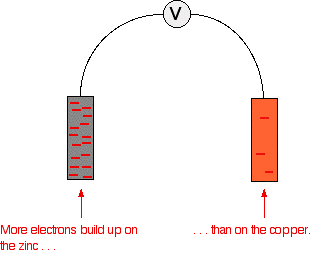
The negative sign of the zinc E° value shows that it releases electrons more readily than hydrogen does. The positive sign of the copper E° shows that it releases electrons less readily than hydrogen. That means that you can compare any two equilibria directly. For example, in this case you can see that the zinc releases electrons more readily than the copper does - the position of the zinc equilibrium lies further to the left than the copper equilibrium. Stripping everything else out of the diagram, and looking only at the build up of electrons on the two pieces of metal:
Obviously, the voltmeter will show that the zinc is the negative electrode, and copper is the (relatively) positive one. It will register a voltage showing the difference between them. | ||
|
Note: It is very simple to calculate this voltage from the E° values. If you are interested in doing this, then you may like to look at my chemistry calculations book. It is impossible for me to include these calculations on this site without upsetting my publishers! | ||
|
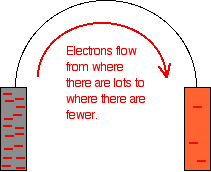
Removing the voltmeter The high resistance of the voltmeter is deliberately designed to stop any current flow in the circuit. What happens if you remove the voltmeter and replace it with a bit of wire? Electrons will flow from where there are a lot of them (on the zinc) to where there are fewer (on the copper). The movement of the electrons is an electrical current. | ||
|
Important: In chemistry, you should always think in terms of electron flow, and never in terms of current flow. The problem is that conventionally in physics current flows in the opposite direction to the electrons. That's totally silly! Avoid the problem. | ||
|
The effect of this on the equilibria These are just simple equilibria, and you can apply Le Chatelier's Principle to them. | ||
|
Note: If you are unsure about Le Chatelier's Principle, then you must follow this link. You don't need to read the whole page - just the beginning. Use the BACK button on your browser to return quickly to this page. | ||
|
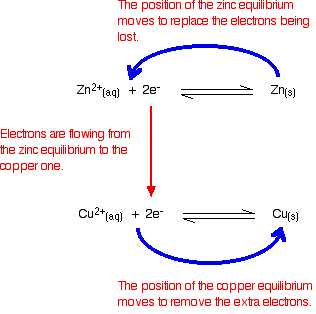
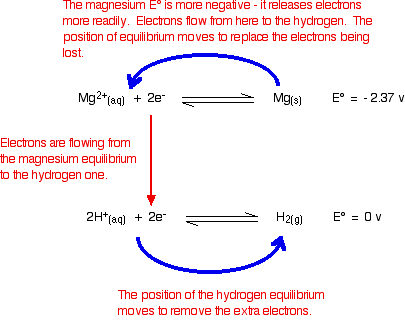
Electrons are flowing away from the zinc equilibrium. According to Le Chatelier's Principle, the position of equilibrium will move to replace the lost electrons. Electrons are being dumped onto the piece of copper in the copper equilibrium. According to Le Chatelier's Principle, the position of equilibrium will move to remove these extra electrons.
If electrons continue to flow, the positions of equilibrium keep on shifting. The two equilibria essentially turn into two one-way reactions. The zinc continues to ionise, and the copper(II) ions keep on picking up electrons.
Taking the apparatus as a whole, there is a chemical reaction going on in which zinc is going into solution as zinc ions, and is giving electrons to copper(II) ions to turn them into metallic copper. Relating this to a test tube reaction This is exactly the same reaction that occurs when you drop a piece of zinc into some copper(II) sulphate solution. The blue colour of the solution fades as the copper(II) ions are converted into brown copper metal. The final solution contains zinc sulphate. (The sulphate ions are spectator ions.) You can add the two electron-half-equations above to give the overall ionic equation for the reaction.
The only difference in this case is that the zinc gives the electrons directly to the copper(II) ions rather than the electrons having to travel along a bit of wire first. The test tube reaction happens because of the relative tendency of the zinc and copper to lose electrons to form ions. You can find out this relative tendency by looking at the E° values. That means that any redox reaction could be discussed in a similar way. The rest of the examples on this page illustrate this. The reaction between copper and silver nitrate solution The reaction in a test tube If you hang a coil of copper wire in some colourless silver nitrate solution, the copper gets covered in silver - partly as a grey fur, and partly as delicate crystals. The solution turns blue. Building the ionic equation from the two half-equations:
| ||
|
Note: If you don't understand why the second equation is multiplied by 2, you really must read about building ionic equations from electron-half-equations. Use the BACK button on your browser to return to this page. | ||
|
Working from the redox potentials How does this relate to the E° values for copper and silver?
You can see that both of these E° values are positive. Neither copper nor silver produce ions and release electrons as easily as hydrogen does. However, of the two, copper releases electrons more readily. In a cell, the copper would have the greater build up of electrons, and be the negative electrode. If the copper and silver were connected by a bit of wire, electrons would flow from the copper to the silver. That, of course, will upset the two equilibria:
Electrons will continue to flow and the two equilibria will again turn into one-way reactions to give the electron-half-equations we've just used to build the ionic equation. Showing that again:
| ||
|
Note: People sometimes worry whether the fact that one of the equations has to be multiplied by two affects the argument in any way. It doesn't! It makes no difference whatsoever to any stage of the explanation in terms of shifts in the positions of the two equilibria. | ||
|
A useful "rule of thumb" Whenever you link two of these equilibria together (either via a bit of wire, or by allowing one of the substances to give electrons directly to another one in a test tube):
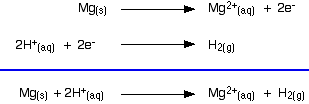
It sounds simple, but that is the key to using E° values in most of the situations you will come across. The reaction between magnesium and dilute sulphuric acid The reaction in a test tube Magnesium reacts with dilute sulphuric acid to give hydrogen and a colourless solution containing magnesium sulphate. Is this what you would expect from the E° values? Working from the redox potentials Putting all the argument onto one diagram:
The two equilibria become one-way reactions which you can use to build the ionic equation:
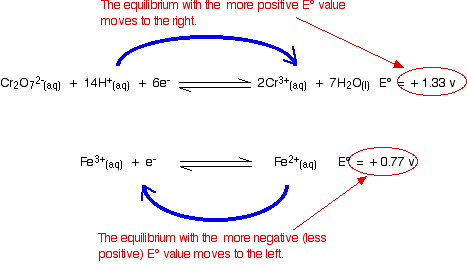
Using potassium dichromate(VI) as an oxidising agent The reaction in a test tube Potassium dichromate(VI) acidified with dilute sulphuric acid oxidises iron(II) ions to iron(III) ions. The orange solution containing the dichromate(VI) ions turns green as chromium(III) ions are formed. How does this relate to the E° values?
| ||
|
Warning! Those of you who have come across other ways of working with E° values may have learnt rules which force you to write the equilibria down in a particular order. I have deliberately written this example down so that it disobeys these rules! This is to show that these rules are completely unnecessary. All you have to do is remember that the more negative (less positive) equilibrium will shift to the left, and the other one to the right. | ||
|
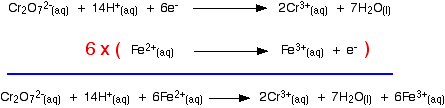
Building the ionic equation then works like this:
Coming up next The final page in the sequence simply expands on this one, and looks at how you can use E° values to predict whether or not redox reactions are feasible. It would be a good idea to be fairly confident about the present page before you went on to the final one.
To the final page on electrode potentials . . . To the redox equilibria menu . . . © Jim Clark 2002 (modified May 2013) |
||